Draw a Diagram of the Unit Circle Chegg

Radians

Radians are another way of measuring angles, and the measure of an angle can be converted between degrees and radians.

Learning Objectives

Explicate the definition of radians in terms of arc length of a unit of measurement circle and use this to convert between degrees and radians

Key Takeaways

Key Points

• One radian is the measure of the cardinal bending of a circle such that the length of the arc is equal to the radius
  of the circumvolve.
• A total revolution of a circle ([latex]360^{\circ}[/latex]) equals [latex]2\pi~\mathrm{radians}[/latex]. This means that [latex]\displaystyle{ ane\text{ radian} = \frac{180^{\circ}}{\pi} }[/latex]. [latex][/latex]
• The formula used to convert between radians and degrees is [latex]\displaystyle{ \text{bending in degrees} = \text{angle in radians} \cdot \frac{180^\circ}{\pi} }[/latex].
• The radian measure of an angle is the ratio of the length of the arc to the radius of the circle [latex]\displaystyle{ \left(\theta = \frac{southward}{r}\correct) }[/latex]. In other words, if [latex]due south[/latex] is the length of an arc of a circle, and [latex]r[/latex] is the radius of the circle, so the central angle containing that arc measures radians.

Central Terms

• arc: A continuous part of the circumference of a circle.
• circumference: The length of a line that bounds a circumvolve.
• radian: The standard unit of measurement used to measure angles in mathematics. The measure of a central angle of a circle that intercepts an arc equal in length to the radius of that circle.

Introduction to Radians

Remember that dividing a circle into 360 parts creates the degree measurement. This is an capricious measurement, and we may cull other means to divide a circle. To find some other unit, call up of the process of drawing a circle. Imagine that you stop before the circumvolve is completed. The portion that you drew is referred to equally an arc. An arc may be a portion of a full circle, a full circle, or more than a full circle, represented by more than one full rotation. The length of the arc around an unabridged circle is called the circumference of that circle.

The circumference of a circle is

[latex]C = 2 \pi r[/latex]

If we divide both sides of this equation by [latex]r[/latex], nosotros create the ratio of the circumference, which is always [latex]2\pi[/latex] to the radius, regardless of the length of the radius. So the circumference of any circle is [latex]2\pi \approx half-dozen.28[/latex] times the length of the radius. That means that if we took a string every bit long as the radius and used it to measure sequent lengths around the circumference, at that place would exist room for six full string-lengths and a fiddling more than than a quarter of a seventh, as shown in the diagram below.

The circumference of a circle compared to the radius: The circumference of a circle is a little more than than 6 times the length of the radius.

This brings us to our new angle measure. The radian is the standard unit used to measure angles in mathematics. One radian is the measure out of a central angle of a circle that intercepts an arc equal in length to the radius of that circle.

I radian: The angle [latex]t[/latex] sweeps out a measure of one radian. Annotation that the length of the intercepted arc is the same equally the length of the radius of the circumvolve.

Considering the total circumference of a circumvolve equals [latex]ii\pi[/latex] times the radius, a full circular rotation is [latex]2\pi[/latex] radians.

Radians in a circle: An arc of a circumvolve with the same length every bit the radius of that circumvolve corresponds to an angle of 1 radian. A full circle corresponds to an angle of [latex]two\pi[/latex] radians; this ways that[latex]2\pi[/latex] radians is the same every bit [latex]360^\circ[/latex].

Note that when an bending is described without a specific unit, it refers to radian measure. For instance, an angle measure of 3 indicates 3 radians. In fact, radian measure is dimensionless, since it is the quotient of a length (circumference) divided by a length (radius), and the length units cancel. Yous may sometimes meet radians represented by the symbol [latex]\text{rad}[/latex].

Comparing Radians to Degrees

Since nosotros now know that the full range of a circle can exist represented by either 360 degrees or [latex]2\pi[/latex] radians, we can conclude the following:

[latex]\displaystyle{ \begin{marshal} ii\pi \text{ radians} &= 360^{\circ} \\ 1\text{ radian} &= \frac{360^{\circ}}{2\pi} \\ 1\text{ radian} &= \frac{180^{\circ}}{\pi} \end{align}}[/latex]

As stated, one radian is equal to [latex]\displaystyle{ \frac{180^{\circ}}{\pi} }[/latex] degrees, or merely under 57.3 degrees ([latex]57.three^{\circ}[/latex]). Thus, to catechumen from radians to degrees, we can multiply by [latex]\displaystyle{ \frac{180^\circ}{\pi} }[/latex]:

[latex]\displaystyle{ \text{angle in degrees} = \text{bending in radians} \cdot \frac{180^\circ}{\pi} }[/latex]

A unit of measurement circle is a circle with a radius of i, and it is used to show sure mutual angles.

Unit circle: Commonly encountered angles measured in radians and degrees.

Example

Convert an angle measuring [latex]\displaystyle{ \frac{\pi}{9} }[/latex] radians to degrees.

Substitute the angle in radians into the above formula:

[latex]\displaystyle{ \begin{marshal} \text{angle in degrees} &= \text{angle in radians} \cdot \frac{180^\circ}{\pi} \\ \text{angle in degrees} &= \frac{\pi}{nine} \cdot \frac{180^\circ}{\pi} \\ &=\frac{180^{\circ}}{9} \\ &= 20^{\circ} \end{marshal} }[/latex]

Thus we take [latex]\displaystyle{ \frac{\pi}{ix} \text{ radians} = 20^{\circ} }[/latex].

Measuring an Angle in Radians

An arc length [latex]due south[/latex] is the length of the curve along the arc. Just equally the full circumference of a circle always has a abiding ratio to the radius, the arc length produced past any given bending likewise has a constant relation to the radius, regardless of the length of the radius.

This ratio, chosen the radian measure, is the same regardless of the radius of the circle—it depends only on the angle. This property allows united states to define a measure of any angle as the ratio of the arc length [latex]south[/latex] to the radius [latex]r[/latex].

[latex]\displaystyle{ \brainstorm{align} s &= r \theta \\ \theta &= \frac{southward}{r} \end{align} }[/latex]

Measuring radians: (a) In an angle of one radian; the arc lengths equals the radius [latex]r[/latex]. (b) An angle of two radians has an arc length [latex]southward=2r[/latex]. (c) A full revolution is [latex]2\pi[/latex], or nigh 6.28 radians.

Example

What is the measure of a given angle in radians if its arc length is [latex]4 \pi[/latex], and the radius has length [latex][/latex]12?

Substitute the values [latex]s = 4\pi[/latex] and [latex]r = 12[/latex] into the angle formula:

[latex]\displaystyle{ \begin{marshal} \theta &= \frac{s}{r} \\ & = \frac{four\pi}{12} \\ &= \frac{\pi}{3} \\ &= \frac{1}{iii}\pi \finish{marshal} }[/latex]

The bending has a measure of [latex]\displaystyle{\frac{1}{3}\pi}[/latex] radians.

Defining Trigonometric Functions on the Unit Circle

Identifying points on a unit circumvolve allows ane to utilise trigonometric functions to any angle.

Learning Objectives

Use right triangles drawn in the unit of measurement circle to define the trigonometric functions for whatsoever bending

Cardinal Takeaways

Primal Points

• The [latex]x[/latex]– and [latex]y[/latex]-coordinates at a point on the unit circle given by an angle [latex]t[/latex] are divers by the functions [latex]x = \cos t[/latex] and [latex]y = \sin t[/latex].
• Although the tangent office is not indicated past the unit circle, we tin can employ the formula [latex]\displaystyle{\tan t = \frac{\sin t}{\cos t}}[/latex] to observe the tangent of any angle identified.
• Using the unit of measurement circle, nosotros are able to apply trigonometric functions to any angle, including those greater than [latex]ninety^{\circ}[/latex].
• The unit of measurement circumvolve demonstrates the periodicity of trigonometric functions past showing that they result in a repeated gear up of values at regular intervals.

Key Terms

• periodicity: The quality of a function with a repeated set of values at regular intervals.
• unit of measurement circle: A circle centered at the origin with radius one.
• quadrants: The four quarters of a coordinate plane, formed by the [latex]x[/latex]– and [latex]y[/latex]-axes.

Trigonometric Functions and the Unit Circle

Nosotros have already divers the trigonometric functions in terms of right triangles. In this section, we volition redefine them in terms of the unit circle. Call up that a unit circle is a circle centered at the origin with radius ane. The angle [latex]t[/latex] (in radians ) forms an arc of length [latex]south[/latex].

The x- and y-axes separate the coordinate plane (and the unit circle, since it is centered at the origin) into four quarters called quadrants. We label these quadrants to mimic the direction a positive angle would sweep. The four quadrants are labeled I, II, Iii, and IV.

For whatever angle [latex]t[/latex], nosotros can label the intersection of its side and the unit circle by its coordinates, [latex](10, y)[/latex]. The coordinates [latex]x[/latex] and [latex]y[/latex] will be the outputs of the trigonometric functions [latex]f(t) = \cos t[/latex] and [latex]f(t) = \sin t[/latex], respectively. This means:

[latex]\displaystyle{ \brainstorm{align} ten &= \cos t \\ ​y &= \sin t \terminate{marshal} }[/latex]

The diagram of the unit of measurement circle illustrates these coordinates.

Unit of measurement circumvolve: Coordinates of a point on a unit of measurement circle where the central angle is [latex]t[/latex] radians.

Note that the values of [latex]x[/latex] and [latex]y[/latex] are given by the lengths of the two triangle legs that are colored red. This is a right triangle, and yous can encounter how the lengths of these two sides (and the values of [latex]x[/latex] and [latex]y[/latex]) are given by trigonometric functions of [latex]t[/latex].

For an example of how this applies, consider the diagram showing the bespeak with coordinates [latex]\displaystyle{\left(-\frac{\sqrt2}{2}, \frac{\sqrt2}{ii}\right) }[/latex] on a unit circle.

Point on a unit circle: The point [latex]\displaystyle{ \left(-\frac{\sqrt2}{2}, \frac{\sqrt2}{ii}\right) }[/latex] on a unit circle.

We know that, for any indicate on a unit of measurement circumvolve, the [latex]x[/latex]-coordinate is [latex]\cos t[/latex] and the [latex]y[/latex]-coordinate is [latex]\sin t[/latex]. Applying this, we can identify that [latex]\displaystyle{\cos t = -\frac{\sqrt2}{ii}}[/latex] and  [latex]\displaystyle{\sin t = -\frac{\sqrt2}{2}}[/latex] for the angle [latex]t[/latex] in the diagram.

Recall that [latex]\displaystyle{\tan t = \frac{\sin t}{\cos t}}[/latex].  Applying this formula, nosotros tin can discover the tangent of any angle identified past a unit circle besides. For the angle [latex]t[/latex] identified in the diagram  of the unit circle showing the point [latex]\displaystyle{\left(-\frac{\sqrt2}{ii}, \frac{\sqrt2}{2}\right)}[/latex], the tangent is:

[latex]\displaystyle{\begin{align}\tan t &= \frac{\sin t}{\cos t} \\&= \frac{-\frac{\sqrt2}{ii}}{-\frac{\sqrt2}{2}} \\&= i\end{align}}[/latex]

We have previously discussed trigonometric functions as they utilise to correct triangles. This allowed us to make observations almost the angles and sides of right triangles, only these observations were limited to angles with measures less than [latex]90^{\circ}[/latex]. Using the unit circumvolve, we are able to use trigonometric functions to angles greater than [latex]90^{\circ}[/latex].

Further Consideration of the Unit Circle

The coordinates of sure points on the unit circle and the the measure of each angle in radians and degrees are shown in the unit of measurement circumvolve coordinates diagram. This diagram allows i to make observations most each of these angles using trigonometric functions.

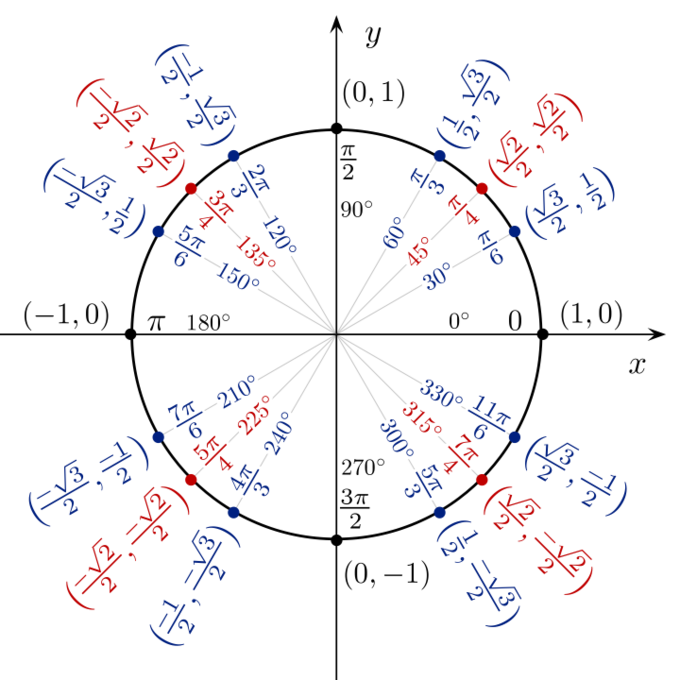
Unit circle coordinates: The unit of measurement circumvolve, showing coordinates and angle measures of certain points.

We can find the coordinates of whatsoever point on the unit of measurement circle. Given any angle [latex]t[/latex], nosotros can notice the [latex]x[/latex]– or [latex]y[/latex]-coordinate at that indicate using [latex]x = \text{cos } t[/latex]  and [latex]y = \text{sin } t[/latex].

The unit of measurement circumvolve demonstrates the periodicity of trigonometric functions. Periodicity refers to the way trigonometric functions outcome in a repeated gear up of values at regular intervals. Have a look at the [latex]x[/latex]-values of the coordinates in the unit circumvolve higher up for values of [latex]t[/latex] from [latex]0[/latex] to [latex]two{\pi}[/latex]:

[latex]{one, \frac{\sqrt{3}}{2}, \frac{\sqrt{2}}{2}, \frac{1}{2}, 0, -\frac{one}{2}, -\frac{\sqrt{two}}{2}, -\frac{\sqrt{three}}{2}, -1, -\frac{\sqrt{3}}{2}, -\frac{\sqrt{2}}{ii}, -\frac{1}{two}, 0, \frac{1}{2}, \frac{\sqrt{2}}{2}, \frac{\sqrt{3}}{ii}, ane}[/latex]

Nosotros tin identify a design in these numbers, which fluctuate between [latex]-1[/latex] and [latex]i[/latex]. Note that this pattern will repeat for higher values of [latex]t[/latex]. Recall that these [latex]x[/latex]-values correspond to [latex]\cos t[/latex]. This is an indication of the periodicity of the cosine part.

Example

Solve [latex]\displaystyle{ \sin{ \left(\frac{seven\pi}{six}\right) } }[/latex].

Information technology seems similar this would be complicated to work out. Even so, notice that the unit circumvolve diagram shows the coordinates at [latex]\displaystyle{ t = \frac{vii\pi}{six} }[/latex]. Since the [latex]y[/latex]-coordinate corresponds to [latex]\sin t[/latex], we can identify that

[latex]\displaystyle{\sin{ \left(\frac{7\pi}{6}\right)} = -\frac{1}{ii} }[/latex]

Special Angles

The unit circumvolve and a set up of rules can exist used to recollect the values of trigonometric functions of special angles.

Learning Objectives

Explicate how the backdrop of sine, cosine, and tangent and their signs in each quadrant give their values for each of the special angles

Key Takeaways

Key Points

• The trigonometric functions for the angles in the unit of measurement circle can exist memorized and recalled using a fix of rules.
• The sign on a trigonometric role depends on the quadrant that the angle falls in, and the mnemonic phrase "A Smart Trig Course" is used to identify which functions are positive in which quadrant.
• Reference angles in quadrant Iare used to identify which value any angle in quadrants II, III, or IV volition take. A reference angle forms the same bending with the [latex]x[/latex]-axis as the bending in question.
• Only the sine and cosine functions for special angles are included in the unit circle. However, since tangent is derived from sine and cosine, it tin can be calculated for whatever of the special angles.

Key Terms

• special angle: An angle that is a multiple of 30 or 45 degrees; trigonometric functions are easily written at these angles.

Trigonometric Functions of Special Angles

Call up that certain angles and their coordinates, which correspond to [latex]x = \cos t[/latex] and [latex]y = \sin t[/latex] for a given angle [latex]t[/latex], can be identified on the unit circle.

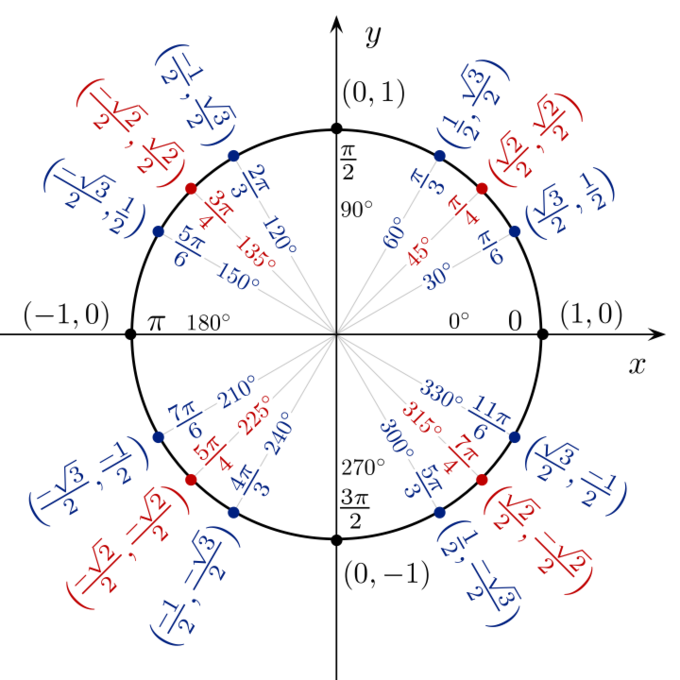
Unit circle: Special angles and their coordinates are identified on the unit circumvolve.

The angles identified on the unit of measurement circumvolve above are called special angles; multiples of [latex]\pi[/latex], [latex]\frac{\pi}{2}[/latex], [latex][/latex][latex]\frac{\pi}{3}[/latex], [latex]\frac{\pi}{4}[/latex], and [latex]\frac{\pi}{6}[/latex] ([latex]180^\circ[/latex][latex][/latex], [latex]90^\circ[/latex], [latex]60^\circ[/latex],  [latex]45^\circ[/latex], and [latex]xxx^\circ[/latex]). These have relatively unproblematic expressions. Such simple expressions mostly practise not exist for other angles. Some examples of the algebraic expressions for the sines of special angles are:

[latex]\displaystyle{ \begin{align} \sin{\left( 0^{\circ} \right)} &= 0 \\ \sin{\left( 30^{\circ} \right)} &= \frac{1}{2} \\ \sin{\left( 45^{\circ} \right)} &= \frac{\sqrt{2}}{ii} \\ \sin{\left( 60^{\circ} \correct)} &= \frac{\sqrt{iii}}{2} \\ \sin{\left( 90^{\circ} \right)} &= 1 \\ \end{align} }[/latex]

The expressions for the cosine functions of these special angles are also elementary.

Note that while simply sine and cosine are defined directly by the unit circle, tangent tin can be defined equally a quotient involving these ii:

[latex]\displaystyle{ \tan t = \frac{\sin t}{\cos t} }[/latex]

Tangent functions also have uncomplicated expressions for each of the special angles.

We can observe this trend through an example. Let'south find the tangent of [latex]60^{\circ}[/latex].

First, we tin identify from the unit of measurement circle that:

[latex]\displaystyle{ \brainstorm{marshal} \sin{ \left(60^{\circ}\right) } &= \frac{\sqrt{3}}{2} \\ \cos{ \left(60^{\circ}\right) } &= \frac{i}{ii} \end{align} }[/latex]

We tin can easily calculate the tangent:

[latex]\displaystyle{ \begin{align} \tan{\left(60^{\circ}\right)} &= \frac{\sin{\left(60^{\circ}\right)}}{\cos{\left(60^{\circ}\right)}} \\ &= \frac{\frac{\sqrt{3}}{2}}{\frac{1}{ii}} \\ &= \frac{\sqrt{3}}{2} \cdot \frac{two}{1} \\ &= \sqrt{iii} \cease{marshal} }[/latex]

Memorizing Trigonometric Functions

An agreement of the unit circle and the ability to apace solve trigonometric functions for sure angles is very useful in the field of mathematics. Applying rules and shortcuts associated with the unit of measurement circle allows y'all to solve trigonometric functions quickly. The following are some rules to help y'all quickly solve such problems.

Signs of Trigonometric Functions

The sign of a trigonometric function depends on the quadrant that the angle falls in. To help remember which of the trigonometric functions are positive in each quadrant, nosotros tin can use the mnemonic phrase "A Smart Trig Class." Each of the four words in the phrase corresponds to 1 of the iv quadrants, starting with quadrant I and rotating counterclockwise. In quadrant I, which is "A," all of the trigonometric functions are positive. In quadrant Ii, "Smart," only sine is positive. In quadrant III, "Trig," only tangent is positive. Finally, in quadrant IV, "Course," merely cosine is positive.

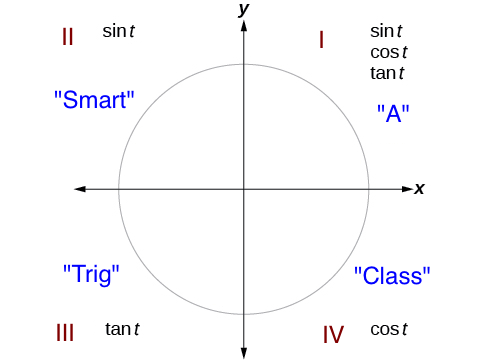
Sign rules for trigonometric functions: The trigonometric functions are each listed in the quadrants in which they are positive.

Identifying Values Using Reference Angles

Take a close look at the unit circumvolve, and note that [latex]\sin t[/latex] and [latex]\cos t[/latex] take certain values every bit they fluctuate between [latex]-ane[/latex] and [latex]ane[/latex]. You will observe that they accept on the value of nil, as well as the positive and negative values of iii particular numbers: [latex]\displaystyle{\frac{\sqrt{three}}{2}}[/latex], [latex]\displaystyle{\frac{\sqrt{2}}{2}}[/latex], and [latex]\displaystyle{\frac{ane}{two}}[/latex]. Identifying reference angles will aid us identify a pattern in these values.

Reference angles in quadrant I are used to place which value any angle in quadrants Two, 3, or IV will take. This means that we only demand to memorize the sine and cosine of 3 angles in quadrant I: [latex]thirty^{\circ}[/latex], [latex]45^{\circ}[/latex], and [latex]sixty^{\circ}[/latex].

For any given angle in the beginning quadrant, there is an angle in the 2nd quadrant with the same sine value. Because the sine value is the [latex]y[/latex]-coordinate on the unit of measurement circle, the other angle with the same sine will share the same [latex]y[/latex]-value, but accept the contrary [latex]ten[/latex]-value. Therefore, its cosine value will be the opposite of the kickoff angle'south cosine value.

As well, there will exist an angle in the fourth quadrant with the same cosine equally the original angle. The angle with the same cosine will share the same [latex]x[/latex]-value only will accept the contrary [latex]y[/latex]-value. Therefore, its sine value volition exist the opposite of the original angle's sine value.

As shown in the diagrams below, angle [latex]\blastoff[/latex] has the aforementioned sine value equally angle [latex]t[/latex]; the cosine values are opposites. Bending [latex]\beta[/latex] has the same cosine value as bending [latex]t[/latex]; the sine values are opposites.

[latex]\displaystyle{ \begin{marshal} \sin t = \sin \alpha \quad &\text{and} \quad \cos t = -\cos \alpha \\ \sin t = -\sin \beta \quad &\text{and} \quad \cos t = \cos \beta \stop{align} }[/latex]

Reference angles: In the left figure, [latex]t[/latex] is the reference angle for [latex]\alpha[/latex]. In the right figure, [latex]t[/latex] is the reference bending for [latex]\beta[/latex].

Recall that an angle'south reference angle is the acute bending, [latex]t[/latex], formed by the final side of the bending [latex]t[/latex] and the horizontal axis. A reference angle is always an angle between [latex]0[/latex] and [latex]90^{\circ}[/latex], or [latex]0[/latex] and [latex]\displaystyle{\frac{\pi}{2}}[/latex] radians. For whatsoever bending in quadrants II, 3, or IV, in that location is a reference angle in quadrant I.

Reference angles in each quadrant: For whatever angle in quadrants Two, 3, or IV, there is a reference bending in quadrant I.

Thus, in order to recall any sine or cosine of a special bending, yous need to be able to identify its angle with the [latex]x[/latex]-axis in order to compare it to a reference angle. You volition then identify and utilise the advisable sign for that trigonometric part in that quadrant.

These are the steps for finding a reference angle for whatever angle between [latex]0[/latex] and [latex]2\pi[/latex]:

1. An angle in the showtime quadrant is its own reference angle.
2. For an angle in the second or 3rd quadrant, the reference angle is [latex]|\pi - t|[/latex] or [latex]|180^{\circ} - t|[/latex].
3. For an angle in the fourth quadrant, the reference angle is [latex]2\pi - t[/latex] or [latex]360^{\circ} - t[/latex]. If an angle is less than [latex]0[/latex] or greater than [latex]2\pi[/latex], add or subtract [latex]2\pi[/latex] as many times every bit needed to discover an equivalent angle between [latex]0[/latex] and [latex]ii\pi[/latex].

Since tangent functions are derived from sine and cosine, the tangent tin can exist calculated for any of the special angles past first finding the values for sine or cosine.

Example

Notice [latex]\tan (225^{\circ})[/latex], applying the rules in a higher place.

First, note that [latex]225^{\circ}[/latex] falls in the third quadrant:

Angle [latex]225^{\circ}[/latex] on a unit circle: The angle [latex]225^{\circ}[/latex]falls in quadrant III.

Subtract [latex]225^{\circ}[/latex] from [latex]180^{\circ}[/latex] to identify the reference angle:

[latex]\displaystyle{ \begin{align} \left| 180^{\circ} - 225^{\circ} \right| &= \left|-45^{\circ} \right| \\ &= 45^{\circ} \finish{marshal} }[/latex]

In other words, [latex]225^{\circ}[/latex] falls [latex]45^{\circ}[/latex] from the [latex]x[/latex]-axis. The reference angle is [latex]45^{\circ}[/latex].

Recall that

[latex]\displaystyle{\sin{ \left(45^{\circ}\right)} = \frac{\sqrt{2}}{2} }[/latex]

However, the rules described to a higher place tell us that the sine of an bending in the third quadrant is negative. So we have

[latex]\displaystyle{\sin{ \left(225^{\circ}\right)} = -\frac{\sqrt{2}}{2} }[/latex]

Following the same process for cosine, we tin can identify that

[latex]\displaystyle{ \cos{ \left(225^{\circ}\right)} = -\frac{\sqrt{2}}{2} }[/latex]

Nosotros can discover [latex]\tan (225^{\circ})[/latex] by dividing [latex]\sin (225^{\circ})[/latex] by [latex]\cos (225^{\circ})[/latex]:

[latex]\displaystyle{ \begin{align} \tan{ \left(225^{\circ}\correct)} &= \frac{\sin(225^{\circ})}{\cos (225^{\circ})} \\ &= \frac{-\frac{\sqrt{2}}{two}}{-\frac{\sqrt{ii}}{2}} \\ &= -\frac{\sqrt{2}}{2} \cdot -\frac{2}{\sqrt{two}} \\ &= 1 \terminate{align} }[/latex]

Sine and Cosine every bit Functions

The functions sine and cosine tin be graphed using values from the unit circle, and certain characteristics tin can exist observed in both graphs.

Learning Objectives

Depict the characteristics of the graphs of sine and cosine

Key Takeaways

Key Points

• Both the sine function [latex](y = \sin x)[/latex] and cosine role [latex](y = \cos 10)[/latex] tin be graphed past plotting points derived from the unit circle, with each [latex]x[/latex]-coordinate being an angle in radians and the [latex]y[/latex]-coordinate existence the corresponding value of the office at that angle.
• Sine and cosine are periodic functions with a period of [latex]2\pi[/latex].
• Both sine and cosine take a domain of [latex](-\infty, \infty)[/latex] and a range of [latex][-one, ane][/latex].
• The graph of [latex]y = \sin x[/latex] is symmetric about the origin considering it is an odd function, while the graph of [latex]y = \cos x[/latex] is symmetric well-nigh the [latex]y[/latex]-centrality because information technology is an even role.

Key Terms

• flow: An interval containing values that occur repeatedly in a part.
• even function: A continuous ready of [latex]\left(10,f(x)\correct)[/latex] points in which [latex]f(-x) = f(10)[/latex], with symmetry about the [latex]y[/latex]-axis.
• odd function: A continuous set of [latex]\left(x, f(x)\right)[/latex] points in which [latex]f(-10) = -f(10)[/latex], with symmetry virtually the origin.
• periodic part: A continuous gear up of [latex]\left(x,f(x)\correct)[/latex] points that repeats at regular intervals.

Graphing Sine and Cosine Functions

Call up that the sine and cosine functions relate existent number values to the [latex]x[/latex]– and [latex]y[/latex]-coordinates of a indicate on the unit of measurement circumvolve. So what do they wait similar on a graph on a coordinate aeroplane? Allow'due south start with the sine office, [latex]y = \sin ten[/latex]. Nosotros tin create a tabular array of values and utilize them to sketch a graph. Beneath are some of the values for the sine role on a unit circle, with the [latex]x[/latex]-coordinate being the angle in radians and the [latex]y[/latex]-coordinate existence [latex]\sin ten[/latex]:

[latex]\displaystyle{ (0, 0) \quad (\frac{\pi}{vi}, \frac{1}{2}) \quad (\frac{\pi}{4}, \frac{\sqrt{two}}{2}) \quad (\frac{\pi}{3}, \frac{\sqrt{3}}{2}) \quad (\frac{\pi}{two}, ane) \\ (\frac{ii\pi}{3}, \frac{\sqrt{3}}{2}) \quad (\frac{3\pi}{four}, \frac{\sqrt{2}}{2}) \quad (\frac{five\pi}{6}, \frac{1}{ii}) \quad (\pi, 0) }[/latex]

Plotting the points from the table and standing along the [latex]x[/latex]-centrality gives the shape of the sine function.

Graph of the sine function: Graph of points with [latex]10[/latex] coordinates beingness angles in radians, and [latex]y[/latex] coordinates being the function [latex]\sin x[/latex].

Notice how the sine values are positive betwixt [latex]0[/latex] and [latex]\pi[/latex], which correspond to the values of the sine function in quadrants I and Ii on the unit circle, and the sine values are negative between [latex]\pi[/latex] and [latex]ii\pi[/latex], which correspond to the values of the sine function in quadrants III and Iv on the unit circle.

Plotting values of the sine function: The points on the bend [latex]y = \sin x[/latex] correspond to the values of the sine function on the unit circle.

Now let'southward take a similar wait at the cosine function, [latex]f(x) = \sin ten[/latex]. Again, we can create a table of values and use them to sketch a graph. Below are some of the values for the sine function on a unit circle, with the [latex]x[/latex]-coordinate being the angle in radians and the [latex]y[/latex]-coordinate being [latex]\cos x[/latex]:

[latex]\displaystyle{ (0, 1) \quad (\frac{\pi}{6}, \frac{\sqrt{3}}{2}) \quad (\frac{\pi}{4}, \frac{\sqrt{2}}{2}) \quad (\frac{\pi}{3}, \frac{1}{ii}) \quad (\frac{\pi}{ii}, 0) \\ (\frac{two\pi}{3}, -\frac{1}{2}) \quad (\frac{3\pi}{4}, -\frac{\sqrt{two}}{2}) \quad (\frac{5\pi}{6}, -\frac{\sqrt{3}}{ii}) \quad (\pi, -ane) }[/latex]

Every bit with the sine part, we can plots points to create a graph of the cosine part.

Graph of the cosine function: The points on the curve [latex]y = \cos ten[/latex] correspond to the values of the cosine function on the unit circle.

Because we can evaluate the sine and cosine of any real number, both of these functions are defined for all existent numbers. By thinking of the sine and cosine values every bit coordinates of points on a unit circle, it becomes articulate that the range of both functions must be the interval [latex]\left[-1, i \correct][/latex].

Identifying Periodic Functions

In the graphs for both sine and cosine functions, the shape of the graph repeats after [latex]2\pi[/latex], which means the functions are periodic with a period of [latex]2\pi[/latex]. A periodic function is a function with a repeated set of values at regular intervals. Specifically, it is a function for which a specific horizontal shift, [latex]P[/latex], results in a function equal to the original function:

[latex]f(x + P) = f(x)[/latex]

for all values of [latex]x[/latex] in the domain of [latex]f[/latex]. When this occurs, we call the smallest such horizontal shift with [latex]P>0[/latex] the flow of the function. The diagram below shows several periods of the sine and cosine functions.

Periods of the sine and cosine functions: The sine and cosine functions are periodic, significant that a specific horizontal shift, [latex]P[/latex], results in a function equal to the original part:[latex]f(x + P) = f(x)[/latex].

Even and Odd Functions

Looking once again at the sine and cosine functions on a domain centered at the [latex]y[/latex]-axis helps reveal symmetries. As we can encounter in the graph of the sine function, it is symmetric nearly the origin, which indicates that it is an odd role. All along the graph, whatsoever two points with reverse [latex]10[/latex] values also have contrary [latex]y[/latex] values. This is characteristic of an odd function: 2 inputs that are opposites have outputs that are likewise opposites. In other words, if [latex]\sin (-x) = - \sin x[/latex].

Odd symmetry of the sine function: The sine function is odd, meaning it is symmetric about the origin.

The graph of the cosine office shows that it is symmetric about the y-centrality. This indicates that it is an fifty-fifty function. For fifty-fifty functions, any two points with opposite [latex]x[/latex]-values have the same office value. In other words, [latex]\cos (-x) = \cos x[/latex]. Nosotros can see from the graph that this is true by comparing the [latex]y[/latex]-values of the graph at any opposite values of [latex]x[/latex].

Even symmetry of the cosine part: The cosine function is even, meaning it is symmetric virtually the [latex]y[/latex]-axis.

Tangent as a Function

Characteristics of the tangent function tin can be observed in its graph.

Learning Objectives

Describe the characteristics of the graph of the tangent function

Primal Takeaways

Primal Points

• The tangent function is undefined at whatsoever value of [latex]x[/latex] where [latex]\cos x = 0[/latex], and its graph has vertical asymptotes at these [latex]x[/latex] values.
• Tangent is a periodic function with a period of [latex]\pi[/latex].
• The graph of the tangent office is symmetric around the origin, and thus is an odd role.

Key Terms

• periodic part: A continuous set of [latex]\left(x, f(x)\correct)[/latex] points with a set of values that repeats at regular intervals.
• period: An interval containing the minimum fix of values that repeat in a periodic function.
• odd office: An continuous gear up of [latex]\left(10, f(x)\right)[/latex] points in which [latex]f(-ten) = -f(ten)[/latex], and there is symmetry about the origin.
• vertical asymptote: A straight line parallel to the [latex]y[/latex] axis that a curve approaches arbitrarily closely equally the curve goes to infinity.

Graphing the Tangent Function

The tangent function can exist graphed by plotting [latex]\left(x,f(x)\right)[/latex]  points. The shape of the function tin can be created by finding the values of the tangent at special angles. Withal, it is not possible to find the tangent functions for these special angles with the unit of measurement circumvolve. We utilise the formula, [latex]\displaystyle{ \tan ten = \frac{\sin x}{\cos x} }[/latex] to decide the tangent for each value.

We can analyze the graphical behavior of the tangent role past looking at values for some of the special angles. Consider the points below, for which the [latex]x[/latex]-coordinates are angles in radians, and the [latex]y[/latex]-coordinates are [latex]\tan x[/latex]:

[latex]\displaystyle{ (-\frac{\pi}{2}, \text{undefined}) \quad (-\frac{\pi}{iii}, -\sqrt{3}) \quad (-\frac{\pi}{iv}, -1) \quad (-\frac{\pi}{6}, -\frac{\sqrt{three}}{3}) \quad (0, 0) \\ (\frac{\pi}{half-dozen}, \frac{\sqrt{3}}{3}) \quad (\frac{\pi}{4}, ane) \quad (\frac{\pi}{3}, \sqrt{iii}) \quad (\frac{\pi}{2}, \text{undefined}) }[/latex]

Find that [latex]\tan x[/latex] is undefined at [latex]\displaystyle{10 = -\frac{\pi}{2}}[/latex] and [latex]\displaystyle{x = \frac{\pi}{2}}[/latex]. The above points volition help usa depict our graph, merely we demand to make up one's mind how the graph behaves where it is undefined. Allow's consider the last four points. We can place that the values of [latex]y[/latex] are increasing as [latex]x[/latex] increases and approaches [latex]\displaystyle{\frac{\pi}{ii}}[/latex]. Nosotros could consider additional points betwixt [latex]\displaystyle{x=0}[/latex] and [latex]\displaystyle{x = \frac{\pi}{2}}[/latex], and we would run across that this holds. Besides, we can meet that [latex]y[/latex] decreases every bit [latex]x[/latex] approaches [latex]\displaystyle{-\frac{\pi}{2}}[/latex], considering the outputs get smaller and smaller.

Recollect that there are multiple values of [latex]x[/latex] that tin give [latex]\cos 10 = 0[/latex]. At whatever such point, [latex]\tan x[/latex] is undefined because [latex]\displaystyle{\tan x = \frac{\sin x}{\cos ten}}[/latex]. At values where the tangent office is undefined, there are discontinuities in its graph. At these values, the graph of the tangent has vertical asymptotes.

Graph of the tangent function: The tangent function has vertical asymptotes at [latex]\displaystyle{ten = \frac{\pi}{ii}}[/latex] and [latex]\displaystyle{ten = -\frac{\pi}{ii}}[/latex].

Characteristics of the Graph of the Tangent Function

As with the sine and cosine functions, tangent is a periodic function. This means that its values repeat at regular intervals. The period of the tangent part is [latex]\pi[/latex] because the graph repeats itself on [latex]x[/latex]-axis intervals of [latex]yard\pi[/latex], where [latex]k[/latex] is a constant. In the graph of the tangent part on the interval [latex]\displaystyle{-\frac{\pi}{2}}[/latex] to [latex]\displaystyle{\frac{\pi}{2}}[/latex], we tin encounter the behavior of the graph over one complete cycle of the function. If nosotros look at
whatever larger interval, we volition see that the characteristics of the graph repeat.

The graph of the tangent function is symmetric effectually the origin, and thus is an odd role. In other words, [latex]\text{tan}(-x) = - \text{tan } x[/latex] for any value of [latex]x[/latex]. Whatsoever two points with contrary values of [latex]ten[/latex] produce reverse values of [latex]y[/latex]. Nosotros can come across that this is true by considering the [latex]y[/latex] values of the graph at any opposite values of [latex]x[/latex]. Consider [latex]\displaystyle{ten=\frac{\pi}{three}}[/latex] and [latex]\displaystyle{x=-\frac{\pi}{3}}[/latex]. We already determined above that [latex]\displaystyle{\tan (\frac{\pi}{3}) = \sqrt{3}}[/latex], and [latex]\displaystyle{\tan (-\frac{\pi}{iii}) = -\sqrt{3}}[/latex].

Secant and the Trigonometric Cofunctions

Trigonometric functions accept reciprocals that tin be calculated using the unit circle.

Learning Objectives

Calculate values for the trigonometric functions that are the reciprocals of sine, cosine, and tangent

Key Takeaways

Key Points

• The secant function is the reciprocal of the cosine function [latex]\displaystyle{\left(\sec 10 = \frac{1}{\cos ten}\correct)}[/latex]. Information technology tin can be plant for an angle [latex]t[/latex] by using the [latex]10[/latex]-coordinate of  the associated bespeak on the unit circle: [latex]\displaystyle{\sec t = \frac{1}{x}}[/latex].
• The cosecant function is the reciprocal of the sine function [latex]\displaystyle{\left(\csc 10 = \frac{1}{\sin x}\right)}[/latex]. It can be found for an angle [latex]t[/latex] by using the [latex]y[/latex]-coordinate of  the associated point on the unit circumvolve: [latex]\displaystyle{\csc t = \frac{1}{y}}[/latex].
• The cotangent function is the reciprocal of the tangent part [latex]\displaystyle{\left(\cot x = \frac{i}{\tan x} = \frac{\cos t}{\sin t}\right)}[/latex]. Information technology tin can be found for an angle by using the [latex]10[/latex]– and [latex]y[/latex]-coordinates of  the associated point on the unit circle: [latex]\displaystyle{\cot t = \frac{\cos t}{\sin t} = \frac{x}{y}}[/latex].

Key Terms

• secant: The reciprocal of the cosine role
• cosecant: The reciprocal of the sine role
• cotangent: The reciprocal of the tangent function

Introduction to Reciprocal Functions

We have discussed three trigonometric functions: sine, cosine, and tangent. Each of these functions has a reciprocal function, which is divers by the reciprocal of the ratio for the original trigonometric role. Notation that reciprocal functions differ from inverse functions. Inverse functions are a fashion of working backwards, or determining an bending given a trigonometric ratio; they involve working with the same ratios as the original function.

The three reciprocal functions are described below.

Secant

The secant function is the reciprocal of the cosine function, and is abbreviated equally [latex]\sec[/latex].
Information technology can be described as the ratio of the length of the hypotenuse to the length of the adjacent side in a triangle.

[latex]\displaystyle{ \begin{align} \sec x &= \frac{i}{\cos 10} \\ \sec x &= \frac{\text{hypotenuse}}{\text{next}} \end{marshal} }[/latex]

It is easy to summate secant with values in the unit of measurement circumvolve. Recall that for any betoken on the circle, the [latex]x[/latex]-value gives [latex]\cos t[/latex] for the associated bending [latex]t[/latex]. Therefore, the secant function for that bending is

[latex]\displaystyle{\sec t = \frac{1}{x}}[/latex]

Cosecant

The cosecant role is the reciprocal of the sine function, and is abbreviated every bit[latex]\csc[/latex]. It can exist described every bit the ratio of the length of the hypotenuse to the length of the contrary side in a triangle.

[latex]\displaystyle{ \begin{marshal} \csc x &= \frac{1}{\sin x} \\ \csc x &= \frac{\text{hypotenuse}}{\text{opposite}} \terminate{align} }[/latex]

As with secant, cosecant can be calculated with values in the unit circumvolve. Recall that for whatever point on the circle, the [latex]y[/latex]-value gives [latex]\sin t[/latex]. Therefore, the cosecant part for the same angle is

[latex]\displaystyle{\csc t = \frac{1}{y}}[/latex]

Cotangent

The cotangent function is the reciprocal of the tangent function, and is abbreviated as [latex]\cot[/latex]. Information technology tin can be described as the ratio of the length of the adjacent side to the length of the hypotenuse in a triangle.

[latex]\displaystyle{ \begin{align} \cot x &= \frac{1}{\tan x} \\ \cot ten &= \frac{\text{adjacent}}{\text{opposite}} \terminate{align} }[/latex]

Besides annotation that considering [latex]\displaystyle{\tan 10 = \frac{\sin x}{\cos x}}[/latex], its reciprocal is

[latex]\displaystyle{\cot x = \frac{\cos 10}{\sin 10}}[/latex]

Cotangent can also exist calculated with values in the unit of measurement circumvolve. Applying the [latex]x[/latex]– and [latex]y[/latex]-coordinates associated with angle [latex]t[/latex], we accept

[latex]\displaystyle{ \begin{align} \cot t &= \frac{\cos t}{\sin t} \\ \cot t &= \frac{x}{y} \cease{align} }[/latex]

Calculating Reciprocal Functions

We now recognize 6 trigonometric functions that can be calculated using values in the unit of measurement circumvolve. Recall that nosotros used values for the sine and cosine functions to calculate the tangent function. We will follow a like process for the reciprocal functions, referencing the values in the unit circle for our calculations.

For case, let's find the value of [latex]\sec{\left(\frac{\pi}{three}\correct)}[/latex].

Applying [latex]\displaystyle{\sec x = \frac{ane}{\cos x}}[/latex], we tin rewrite this equally:

[latex]\displaystyle{ \sec{\left(\frac{\pi}{three}\right)}= \frac{1}{\cos{\left({\frac{\pi}{three}}\right)}} }[/latex]

From the unit circle, we know that [latex]\displaystyle{\cos{\left({\frac{\pi}{3}}\correct)}= \frac{1}{2}}[/latex]. Using this, the value of [latex]\displaystyle{ \sec{\left(\frac{\pi}{3}\right)}}[/latex] can be institute:

[latex]\displaystyle{ \begin{align} \sec{\left(\frac{\pi}{3}\right)} &= \frac{1}{\frac{i}{2}} \\ &= 2 \end{marshal} }[/latex]

The other reciprocal functions can be solved in a similar manner.

Instance

Use the unit circumvolve to calculate [latex]\sec t[/latex], [latex]\cot t[/latex], and [latex]\csc t[/latex] at the point [latex]\displaystyle{\left(-\frac{\sqrt{three}}{ii}, \frac{ane}{2}\right)}[/latex].

Point on a unit circle: The point [latex]\displaystyle{\left(-\frac{\sqrt{3}}{ii}, \frac{ane}{2}\correct)}[/latex], shown on a unit circumvolve.

Because we know the [latex](x, y)[/latex] coordinates of the bespeak on the unit circle indicated by bending [latex]t[/latex], we can use those coordinates to notice the three functions.

Remember that the [latex]10[/latex]-coordinate gives the value for the cosine function, and the [latex]y[/latex]-coordinate gives the value for the sine function. In other words:

[latex]\displaystyle{ \begin{align} ten &= \cos t \\ &= -\frac{\sqrt{3}}{two} \end{align} }[/latex]

and

[latex]\displaystyle{ \brainstorm{align} y &= \sin t \\ &= \frac{1}{2} \end{align} }[/latex]

Using this information, the values for the reciprocal functions at angle [latex]t[/latex] tin can exist calculated:

[latex]\displaystyle{ \begin{align} \sec t &= \frac{1}{\cos t} \\ &= \frac{1}{x} \\ &= \left(\frac{1}{-\frac{\sqrt{3}}{two}} \right)\\ &= -\frac{2}{\sqrt{iii}} \\ &= \left(-\frac{2}{\sqrt{3}} \cdot \frac{\sqrt{3}}{\sqrt{iii}} \right)\\ &= -\frac{2\sqrt{three}}{3} \end{marshal} }[/latex]

[latex]\displaystyle{ \begin{marshal} \cot t &= \frac{\cos t}{\sin t} \\ &= \frac{x}{y} \\ &= \left(\frac{-\frac{\sqrt{3}}{2}}{\frac{one}{2}}\right) \\ &= \left(-\frac{\sqrt{three}}{two}\cdot \frac{ii}{i} \right) \\ &= -\sqrt{iii} \stop{align} }[/latex]

[latex]\displaystyle{ \brainstorm{align} \csc t &= \frac{1}{\sin t} \\ & = \frac{1}{y} \\ & = \left(\frac{1}{\frac{1}{2}}\right) \\ & = 2 \end{align} }[/latex]

Inverse Trigonometric Functions

Each trigonometric function has an changed function that tin be graphed.

Learning Objectives

Draw the characteristics of the graphs of the changed trigonometric functions, noting their domain and range restrictions

Central Takeaways

Primal Points

• The inverse function of sine is arcsine, which has a domain of [latex]\displaystyle{\left[-\frac{\pi}{2}, \frac{\pi}{two}\right]}[/latex]. In other words, for angles in the interval [latex]\displaystyle{\left[-\frac{\pi}{2}, \frac{\pi}{two}\correct]}[/latex], if [latex]y = \sin x[/latex], then [latex]\arcsin x = \sin^{−ane} ten=y[/latex].
• The inverse office of cosine is arccosine, which has a domain of [latex]\left[0, \pi\right][/latex]. In other words, for angles in the interval [latex]\left[0, \pi\correct][/latex], if [latex]y = \cos 10[/latex], then [latex]\arccos x = \cos^{−1} x=y[/latex].
• The inverse function of tangent is arctangent, which has a domain of [latex]\left(-\frac{\pi}{ii}, \frac{\pi}{2}\right)[/latex]. In other words, for angles in the interval [latex]\left(-\frac{\pi}{2}, \frac{\pi}{2}\correct)[/latex], if [latex]y = \tan x[/latex], then [latex]\arctan x = \tan^{−i} ten=y[/latex].

Key Terms

• changed function: A role that does exactly the opposite of another. Note: [latex]f^{-1}[/latex]
• i-to-one function: A function that never maps distinct elements of its domain to the aforementioned element of its range.

Introduction to Inverse Trigonometric Functions

Inverse trigonometric functions are used to find angles of a triangle if we are given the lengths of the sides. Inverse trigonometric functions can be used to determine what bending would yield a specific sine, cosine, or tangent value.

To use changed trigonometric functions, nosotros need to understand that an inverse trigonometric function "undoes" what the original trigonometric function "does," as is the example with any other part and its inverse.

The inverse of sine is arcsine (denoted [latex]\arcsin[/latex]), the inverse of cosine is arccosine (denoted [latex]\arccos[/latex]), and the inverse of tangent is arctangent (denoted [latex]\arctan[/latex]).

Note that the domain of the inverse function is the range of the original part, and vice versa. An exponent of [latex]-1[/latex] is used to bespeak an changed office. For example, if [latex]f(10) = \sin 10[/latex],then we would write [latex]f^{-i}(x) = \sin^{-1} x[/latex]. Be aware that [latex]\sin^{-1} 10[/latex] does not hateful [latex]\displaystyle{\frac{i}{\sin x}}[/latex]. The reciprocal function is [latex]\displaystyle{\frac{1}{\sin x}}[/latex], which is not the same equally the changed function.

For a one-to-1 role, if [latex]f(a) = b[/latex], so an inverse role would satisfy [latex]f^{-1}(b) = a[/latex]. However, the sine, cosine, and tangent functions are notone-to-one functions. The graph of each function would fail the horizontal line test. In fact, no periodic role can exist one-to-one because each output in its range corresponds to at least one input in every flow, and at that place are an space number of periods. As with other functions that are not i-to-one, we will demand to restrict the domain of each function to yield a new part that is one-to-one. We cull a domain for each function that includes the number [latex]0[/latex].

Sine and cosine functions within restricted domains: (a) The sine role shown on a restricted domain of [latex]\left[-\frac{\pi}{2}, \frac{\pi}{2}\right][/latex]; (b) The cosine role shown on a restricted domain of [latex]\left[0, \pi\correct][/latex].

The graph of the sine function is express to a domain of [latex][-\frac{\pi}{two}, \frac{\pi}{2}][/latex], and the graph of the cosine function limited is to [latex][0, \pi][/latex]. The graph of the tangent function is limited to [latex]\left(-\frac{\pi}{ii}, \frac{\pi}{ii}\right)[/latex].

Tangent function within a restricted domain

The tangent role shown on a restricted domain of [latex]\left(-\frac{\pi}{2}, \frac{\pi}{2}\correct)[/latex].

These choices for the restricted domains are somewhat capricious, simply they have important, helpful characteristics. Each domain includes the origin and some positive values, and near importantly, each results in a 1-to-one function that is invertible. The conventional choice for the restricted domain of the tangent function too has the useful holding that it extends from one vertical asymptote to the next, instead of being divided into pieces by an asymptote.

Definitions of Changed Trigonometric Functions

We can define the inverse trigonometric functions equally follows. Notation the domain and range of each function.

The inverse sine function [latex]y = \sin^{-ane}x [/latex] means [latex]x = \sin y[/latex]. The changed sine function tin also be written [latex]\arcsin x[/latex].

[latex]\displaystyle{y = \sin^{-1}x \quad \text{has domain} \quad \left[-1, i\right] \quad \text{and range} \quad \left[-\frac{\pi}{2}, \frac{\pi}{two}\right]}[/latex]

The inverse cosine function [latex]y = \cos^{-1}ten [/latex] means [latex]ten = \cos y[/latex]. The inverse cosine role tin likewise be written [latex]\arccos ten[/latex].

[latex]\displaystyle{y = \cos^{-1}x \quad \text{has domain} \quad \left[-ane, 1\right] \quad \text{and range} \quad \left[0, \pi\right]}[/latex]

The inverse tangent role [latex]y = \tan^{-one}10[/latex] means [latex]x = \tan y[/latex]. The inverse tangent function can also exist written [latex]\arctan x[/latex].

[latex]\displaystyle{y = \tan^{-1}x \quad \text{has domain} \quad \left(-\infty, \infty\right) \quad \text{and range} \quad \left(-\frac{\pi}{2}, \frac{\pi}{2}\right)}[/latex]

Graphs of Changed Trigonometric Functions

The sine function and changed sine (or arcsine) role: The arcsine function is a reflection of the sine part about the line [latex]y = x[/latex].

To notice the domain and range of inverse trigonometric functions, we switch the domain and range of the original functions.

The cosine office and inverse cosine (or arccosine) function: The arccosine role is a reflection of the cosine part most the line [latex]y = 10[/latex].

Each graph of the inverse trigonometric function is a reflection of the graph of the original function about the line [latex]y = x[/latex].

The tangent function and inverse tangent (or arctangent) function: The arctangent function is a reflection of the tangent function about the line [latex]y = ten[/latex].

Summary

In summary, nosotros tin can state the following relations:

• For angles in the interval [latex]\displaystyle{\left[-\frac{\pi}{2}, \frac{\pi}{2}\correct]}[/latex], if [latex]\sin y = ten[/latex], then [latex]\sin^{−ane} x=y[/latex].
• For angles in the interval [latex]\displaystyle{\left[0, \pi\correct]}[/latex], if [latex]\cos y = ten[/latex], then [latex]\cos^{-one} x = y[/latex].
• For angles in the interval [latex]\displaystyle{\left(-\frac{\pi}{2}, \frac{\pi}{2}\right)}[/latex], if [latex]\tan y = x[/latex], then [latex]\tan^{-1}x = y[/latex].

jonesbecruily.blogspot.com

Source: https://courses.lumenlearning.com/boundless-algebra/chapter/trigonometric-functions-and-the-unit-circle/

Share this post